FORMAT OF THE GRE MATH SECTION
The Math sections consist of three types of questions: Quantitative Comparisons, Standard Multiple Choice, and Graphs. They are designed to test your ability to solve problems, not to test your mathematical knowledge.
Format
Quantitative Comparisons
Standard Multiple Choice
Nonstandard Multiple Choice
Graphs
Numeric Entry
If you like this material, you’ll love the course!
This interactive, comprehensive self-study course presents the equivalent of over 600 pages of printed material, including hundreds of GRE examples, problems, videos, and feedback from GRE experts to your questions. All for only $19.95! Click the link above for a tour of the course.
Similar online programs cost upwards of $699! With more than $680 in savings and quality that can’t be matched, Nova Press is unquestionably the smartest option in GRE prep.
Course Features:
- Ask Questions! Our experts monitor StudyDesk to answer your questions in real-time during business hours or within 24 hours outside of business hours.
- Highly Interactive: You can take notes, view detailed explanations of solutions, and more!
- Videos: More than 500 videos guide you through the concepts and examples.
- Versatile: Access the course from any computer at any time.
- Statistics: Your performance on the exercises is saved, and you may review your performance and check solutions at any time.
- Guarantee: If, at the end of the course, you do not feel sufficiently prepared for the test, you may repeat the course for free — with full access to our experts.
Reviews:
Love this class. I went from not being able to answer any questions to understanding and solving most of the problems. It is still a work in progress, but I am feeling much more prepared for the test now! Great job! – Ana Maria Moreno
This course is very helpful. The instructor reads a few basic principles of mathematics, and then solves a block of questions which require that particular understanding. You can pause each video before the instructor begins and try to solve it on your own and then compare your method and answer to the narrated approach and correct solution. – Vincent Stowell
LEVEL OF DIFFICULTY
GRE math is very similar to SAT math, though surprisingly slightly easier. The mathematical skills tested are very basic: only first year high school algebra and geometry (no proofs). However, this does not mean that the math section is easy. The medium of basic mathematics is chosen so that everyone taking the GRE will be on a fairly even playing field. This way students who majored in math, engineering, or science don’t have an undue advantage over students who majored in humanities. Although the questions require only basic mathematics and all have simple solutions, it can require considerable ingenuity to find the simple solution. If you have taken a course in calculus or another advanced math topic, don’t assume that you will find the math section easy. Other than increasing your mathematical maturity, little you learned in calculus will help on the GRE.
As mentioned above, every GRE math problem has a simple solution, but finding that simple solution may not be easy. The intent of the math section is to test how skilled you are at finding the simple solutions. The premise is that if you spend a lot of time working out long solutions you will not finish as much of the test as students who spot the short, simple solutions. So if you find yourself performing long calculations or applying advanced mathematics — stop. You’re heading in the wrong direction.
SUBSTITUTION
Substitution is a very useful technique for solving GRE math problems. It often reduces hard problems to routine ones. In the substitution method, we choose numbers that have the properties given in the problem and plug them into the answer-choices.
Example: If n is an odd integer, which one of the following is an even integer?
(A) 3n + 2
(B) n/4
(C) 2n + 3
(D) n(n + 3)
(E) nn
We are told that n is an odd integer. So choose an odd integer for n, say, 1 and substitute it into each answer-choice. In Choice (A), 3(1) + 2 = 5, which is not an even integer. So eliminate (A). Next, n/4 = 1/4 is not an even integer — eliminate (B). Next, 2n + 3 = 2(1) + 3 = 5 is not an even integer — eliminate (C). Next, n(n + 3) = 1(1 + 3) = 4 is even and hence the answer is possibly (D). Finally, in Choice (E), the nn = 1(1) = 1, which is not even — eliminate (E). The answer is (D).
When using the substitution method, be sure to check every answer-choice because the number you choose may work for more than one answer-choice. If this does occur, then choose another number and plug it in, and so on, until you have eliminated all but the answer. This may sound like a lot of computing, but the calculations can usually be done in a few seconds.
When substituting in quantitative comparison problems, don’t rely on only positive whole numbers. You must also check negative numbers, fractions, 0, and 1 because they often give results different from those of positive whole numbers. Plug in the numbers 0, 1, 2, -2, and 1/2, in that order.
Example: Determine which of the two expressions below is larger, whether they are equal, or whether there is not enough information to decide. [The answer is (A) if Column A is larger, (B) if Column B is larger, (C) if the columns are equal, and (D) if there is not enough information to decide.]
| Column A | x ≠ 0 | Column B |
| x | xx |
If x = 2, then Column B is 4. In this case, Column B is larger. However, if x = 1, then Column B is 1. In this case, the two columns are equal. Hence, the answer is (D) — not enough information to decide.
Note: If, as above, you get a certain answer when a particular number is substituted and a different answer when another number is substituted (Double Case), then the answer is (D) — not enough information to decide.
DEFINED FUNCTIONS
Defined functions are very common on the GRE, and most students struggle with them. Yet once you get used to them, defined functions can be some of the easiest problems on the test. In this type of problem, you will be given a symbol and a property that defines the symbol.
Example: Define x # y by the equation x # y = xy – y. Then 2 # 3 =
(A) 1
(B) 3
(C) 12
(D) 15
(E) 18
From the above definition, we know that x # y = xy – y. So all we have to do is replace x with 2 and y with 3 in the definition: 2 # 3 = 2(3) – 3 = 3. Hence, the answer is (B).
Example:
| Define x @ y to be xx. |
| Column A | Column B |
| z @ 2 | z @ 3 |
Most students who are unfamiliar with defined functions are unable to solve this problem. Yet it is actually quite easy. By the definition given above, @ merely squares the first term.
So z @ 2 = zz, and z @ 3 = zz. In each case, the result is zz. Hence the two expressions are equal. The answer is (C).
Are you ready for the math section of the GRE?
With our comprehensive book, you can be sure!
Although the GRE math section is difficult, it is very learnable. GRE Math Prep Course presents a thorough analysis of GRE math and introduces numerous analytic techniques that will help you immensely, not only on the GRE but in graduate school as well.
- Comprehensive Review: Twenty-three chapters provide comprehensive review of GRE math.
- Practice: Includes 164 examples and more than 600 exercises! Arranged from easy to medium to hard to very hard.
- Diagnostic Test: The diagnostic test measures your strengths and weaknesses and directs you to areas you need to study more.
- Dual Exercises: These are pairs of similar GRE problems in which only one property is different. They give you insight into how GRE problems are constructed.
- Performance: If your target is a top tier score, this is the book!
Testimonial:
I have tried other brands to help increase my GRE math score, but I found this book by far to be the best. Some of the questions are indeed harder than what you will see on the test which is a good thing as I studied from this book for 4 weeks and my quantitave score improved drastically. After the 4 weeks, I returned to look at some questions in other math prep books and I could do them all, even the difficult ones quiet easily.
You need a good understanding of math concepts, and this book is not for beginners. It will definitely get you into the 700-800 bracket quiet easily.
Pages: 468
ISBN: 1889057952
Purchase GRE Math Prep Course (printed book) ($20.97) (Free shipping within the United States.) (We do not ship outside of the United States.)
Also available as an Amazon Kindle eBook:
GRE Math Prep Course – Kindle eBook
QUANTITATIVE COMPARISONS
Generally, quantitative comparison questions require much less calculating than do multiple-choice questions. But they are trickier.
Substitution is very effective with quantitative comparison problems. But you must plug in all five major types of numbers: positives, negatives, fractions, 0, and 1. Test 0, 1, 2, -2, and 1/2, in that order.
General Principles For Solving Quantitative Comparisons
- You Can Add or Subtract the Same Term (Number) from Both Sides of a Quantitative Comparison Problem.
- You Can Multiply or Divide Both Sides of a Quantitative Comparison Problem by the Same Positive Term (Number). (Caution: This cannot be done if the term can ever be negative or zero.)
You can think of a quantitative comparison problem as an inequality/equation. Your job is to determine whether the correct symbol with which to compare the columns is <, =, >, or that it cannot be determined. Therefore, all the rules that apply to solving inequalities apply to quantitative comparisons. That is, you can always add or subtract the same term to both columns of the problem. If the term is always positive, then you can multiply or divide both columns by it. (The term cannot be negative because it would then invert the inequality. And, of course, it cannot be zero if you are dividing.)
Example:
| Column A | Column B |
| 1/5 + 1/3 + 1/8 | 1/8 + 1/5 + 1/4 |
Don’t solve this problem by adding the fractions in each column; that would be too time consuming — the LCD is 120! Instead, merely subtract 1/5 and 1/8 from both columns:
| Column A | Column B |
| 1/3 | 1/4 |
Now 1/3 is larger than 1/4, so Column A is larger than Column B.
Note: If there are only numbers (i.e., no variables) in a quantitative comparison problem, then “not-enough-information” cannot be the answer. Hence (D), not-enough-information, cannot be the answer to Example 1 above.
- Caution: You Must Be Certain That the Quantity You Are Multiplying or Dividing by Can Never Be Zero or Negative. (There are no restrictions on adding or subtracting.)
The following example illustrates the false results that can occur if you don’t guarantee that the number you are multiplying or dividing by is positive.
| Column A | 0 < x < 1 | Column B |
| xx | x |
Solution (Invalid): Dividing both columns by x yields
| Column A | Column B | |
| x | 1 |
We are given that x < 1, so Column B is larger. But this is a false result because when x = 0, the two original columns are equal:
| Column A | Column B | |
| (0)(0) = 0 | 0 |
Hence, the answer is actually (D), not-enough-information to decide.
- Caution: Don’t Cancel Willy-Nilly.
Some people are tempted to cancel the 4x from both columns of the following problem:
| Column A | Column B | |
| 4x – 6 | 5y – 6 – 4x |
You cannot cancel the 4x’s from both columns because they do not have the same sign. In Column A, 4x is positive. Whereas in Column B, it is negative.
GEOMETRY
One-fourth of the math problems on the GRE involve geometry. (There are no proofs.) Unfortunately, the figures on the GRE are usually not drawn to scale. Hence, in most cases you cannot solve problems or check your work by “eyeballing” the drawing.
Following are some of the basic properties of geometry. You probably know many of them. Memorize any that you do not know.
1. There are 180 degrees in a straight angle.
2. Two angles are supplementary if their angle sum is 180 degrees.
3. Two angles are complementary if their angle sum is 90 degrees.
4. Perpendicular lines meet at right angles.
5. A triangle with two equal sides is called isosceles. The angles opposite the equal sides are called the base angles.
6. The altitude to the base of an isosceles or equilateral triangle bisects the base and bisects the vertex angle.
7. The angle sum of a triangle is 180 degrees.
8. In an equilateral triangle all three sides are equal, and each angle is 60 degrees.
9. The area of a triangle is bh/2, where b is the base and h is the height.
10. In a triangle, the longer side is opposite the larger angle, and vice versa.
11. Two triangles are similar (same shape and usually different size) if their corresponding angles are equal. If two triangles are similar, their corresponding sides are proportional.
12. Two triangles are congruent (identical) if they have the same size and shape.
13. In a triangle, an exterior angle is equal to the sum of its remote interior angles and is therefore greater than either of them.
14. Opposite sides of a parallelogram are both parallel and congruent.
15. The diagonals of a parallelogram bisect each other.
16. If w is the width and l is the length of a rectangle, then its area is A = lw and its perimeter is P = 2w + 2l.
17. The volume of a rectangular solid (a box) is the product of the length, width, and height. The surface area is the sum of the area of the six faces.
18. If the length, width, and height of a rectangular solid (a box) are the same, it is a cube. Its volume is the cube of one of its sides, and its surface area is the sum of the areas of the six faces.
19. A tangent line to a circle intersects the circle at only one point. The radius of the circle is perpendicular to the tangent line at the point of tangency.
20. An angle inscribed in a semicircle is a right angle.
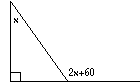
Example: In the figure above, what is the value of x?
(A) 30
(B) 32
(C) 35
(D) 40
(E) 47
Since 2x + 60 is an exterior angle, it is equal to the sum of the remote interior angles. That is, 2x + 60 = x + 90. Solving for x gives x = 30. The answer is (A).
Most geometry problems on the GRE require straightforward calculations. However, some problems measure your insight into the basic rules of geometry. For this type of problem, you should step back and take a “birds-eye” view of the problem. The following example will illustrate.
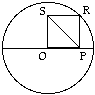
Example: In the figure, O is both the center of the circle with radius 2 and a vertex of the square OPRS. What is the length of diagonal PS?
(A) 1/2
(B) 1
(C) 4
(D) 2
(E) 2/3
The diagonals of a square are equal. Hence, line segment OR (not shown) is equal to SP. Now, OR is a radius of the circle and therefore OR = 2. Hence, SP = 2 as well, and the answer is (D).
COORDINATE GEOMETRY
Distance Formula:
The distance between points (x, y) and (a, b) is given by the following formula:
![]()
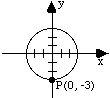
Example: In the figure above, the circle is centered at the origin and passes through point P. Which of the following points does it also pass through?
(A) (3, 3)
(B) ![]()
(C) (2, 6)
(D) (1.5, 1.3)
(E) (-3, 4)
Since the circle is centered at the origin and passes through the point (0,-3), the radius of the circle is 3. Now, if any other point is on the circle, the distance from that point to the center of the circle (the radius) must also be 3. Look at choice (B). Using the distance formula to calculate the distance between ![]() and (0, 0) (the origin) yields
and (0, 0) (the origin) yields
![]()
Hence, ![]() is on the circle, and the answer is (B).
is on the circle, and the answer is (B).
Midpoint Formula:
The midpoint M between points (x, y) and (a, b) is given by
M = ([x + a]/2, [y + b]/2)
In other words, to find the midpoint, simply average the corresponding coordinates of the two points.
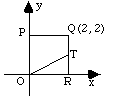
Example: In the figure shown, polygon PQRO is a square and T is the midpoint of side QR. What are the coordinates of T ?
(A) (1, 1)
(B) (1, 2)
(C) (1.5, 1.5)
(D) (2, 1)
(E) (2, 3)
Since point R is on the x-axis, its y-coordinate is 0. Further, since PQRO is a square and the x-coordinate of Q is 2, the x-coordinate of R is also 2. Since T is the midpoint of side QR, the midpoint formula yields
T = ([2 + 2]/2, [2 + 0]/2) = (4/2, 2/2) = (2, 1)
The answer is (D).
Slope Formula:
The slope of a line measures the inclination of the line. By definition, it is the ratio of the vertical change to the horizontal change. The vertical change is called the rise, and the horizontal change is called the run. Thus, the slope is the rise over the run. Given the two points (x, y) and (a, b) the slope is
M = (y – b)/(x – a)

Example: In the figure above, what is the slope of line passing through the two points?
(A) 1/4
(B) 1
(C) 1/2
(D) 3/2
(E) 2
The slope formula yields
m = (4 – 2)/(5 – 1) = 2/4 = 1/2
The answer is (C).
INEQUALITIES
Inequalities are manipulated algebraically the same way as equations with one exception:
Multiplying or dividing both sides of an inequality by a negative number reverses the inequality. That is, if x > y and c < 0, then cx < cy.
Example: For which values of x is 4x + 3 > 6x – 8?
As with equations, our goal is to isolate x on one side:
Subtracting 6x from both sides yields -2x + 3 > -8.
Subtracting 3 from both sides yields -2x > -11.
Dividing both sides by -2 and reversing the inequality yields x < 11/2.
Positive & Negative Numbers
A number greater than 0 is positive. On the number line, positive numbers are to the right of 0. A number less than 0 is negative. On the number line, negative numbers are to the left of 0. Zero is the only number that is neither positive nor negative; it divides the two sets of numbers. On the number line, numbers increase to the right and decrease to the left.
The expression x > y means that x is greater than y. In other words, x is to the right of y on the number line.
We usually have no trouble determining which of two numbers is larger when both are positive or one is positive and the other negative (e.g., 5 > 2 and 3.1 > -2). However, we sometimes hesitate when both numbers are negative (e.g., -2 > -4.5). When in doubt, think of the number line: if one number is to the right of the number, then it is larger.
RATIO & PROPORTION
Ratio
A ratio is simply a fraction. Both of the following notations express the ratio of x to y: x:y, x/y. A ratio compares two numbers. Just as you cannot compare apples and oranges, so too must the numbers you are comparing have the same units. For example, you cannot form the ratio of 2 feet to 4 yards because the two numbers are expressed in different units — feet vs. yards. It is quite common for the GRE to ask for the ratio of two numbers with different units. Before you form any ratio, make sure the two numbers are expressed in the same units.
Example:
| Column A | Column B | ||
| The ratio of 2 miles to 4 miles | The ratio of 2 feet to 4 yards |
Forming the ratio in Column A yields 2 miles/4 miles = 1/2 or 1:2. The ratio in Column B cannot be formed until the numbers are expressed in the same units. Let’s turn the yards into feet. Since there are 3 feet in a yard, 4 yards = 4 x 3 feet = 12 feet. Forming the ratio yields 2 feet/12 feet = 1/6 or 1:6. Hence, Column A is larger.
Proportion
A proportion is simply an equality between two ratios (fractions). For example, the ratio of x to y is equal to the ratio of 3 to 2 is translated as x/y = 3/2. Two variables are directly proportional if one is a constant multiple of the other:
y = kx, where k is a constant.
The above equation shows that as x increases (or decreases) so does y. This simple concept has numerous applications in mathematics. For example, in constant velocity problems, distance is directly proportional to time: d = vt, where v is a constant. Note, sometimes the word directly is suppressed.
Example: If the ratio of y to x is equal to 3 and the sum of y and x is 80, what is the value of y?
(A) -10
(B) -2
(C) 5
(D) 20
(E) 60
Translating “the ratio of y to x is equal to 3” into an equation yields: y/x = 3
Translating “the sum of y and x is 80” into an equation yields: y + x = 80
Solving the first equation for y gives: y = 3x.
Substituting this into the second equation yields
3x + x = 80
4x = 80
x = 20
Hence, y = 3x = 3(20) = 60. The answer is (E).
EXPONENTS & ROOTS
Exponents
There are five rules that govern the behavior of exponents:
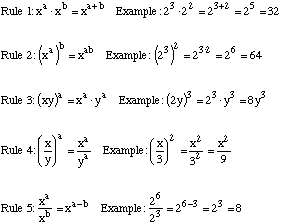
Problems involving these five rules are common on the GRE, and they are often listed as hard problems. However, the process of solving these problems is quite mechanical: simply apply the five rules until they can no longer be applied.
Roots
There are only two rules for roots that you need to know for the GRE:
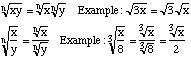
FACTORING
To factor an algebraic expression is to rewrite it as a product of two or more expressions, called factors. In general, any expression on the GRE that can be factored should be factored, and any expression that can be unfactored (multiplied out) should be unfactored.
Distributive Rule
The most basic type of factoring involves the distributive rule:
ax + ay = a(x + y)
For example, 3h + 3k = 3(h + k), and 5xy + 45x = 5xy + 9(5x) = 5x(y + 9). The distributive rule can be generalized to any number of terms. For three terms, it looks like ax + ay + az = a(x + y + z). For example, 2x + 4y + 8 = 2x + 2(2y) + 2(4) = 2(x + 2y + 4).
Example: If x – y = 9, then (x – y/3) – (y – x/3) =
(A) -4
(B) -3
(C) 0
(D) 12
(E) 27
(x – y/3) – (y – x/3) =
x – y/3 – y + x/3 =
4x/3 – 4y/3 =
4(x – y)/3 =
4(9)/3 =
12
The answer is (D).
Difference of Squares
One of the most important formulas on the GRE is the difference of squares:
![]()
Example: If x does not equal -2, then![]()
(A) 2(x – 2)
(B) 2(x – 4)
(C) 8(x + 2)
(D) x – 2
(E) x + 4
In most algebraic expressions involving multiplication or division, you won’t actually multiply or divide, rather you will factor and cancel, as in this problem.
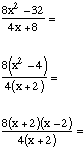
2(x – 2)
The answer is (A).
ALGEBRAIC EXPRESSIONS
A mathematical expression that contains a variable is called an algebraic expression. Some examples of algebraic expressions are 3x – 2y, 2z/y. Two algebraic expressions are called like terms if both the variable parts and the exponents are identical. That is, the only parts of the expressions that can differ are the coefficients. For example, x + y and -7(x + y) are like terms. However, x – y and 2 – y are not like terms.
Adding & Subtracting Algebraic Expressions
Only like terms may be added or subtracted. To add or subtract like terms, merely add or subtract their coefficients:
![]()
You may add or multiply algebraic expressions in any order. This is called the commutative property:
x + y = y + x xy = yx
For example, -2x + 5x = 5x + (-2x) = (5 – 2)x = -3x and (x – y)(-3) = (-3)(x – y) = (-3)x – (-3)y = -3x + 3y.
Caution: the commutative property does not apply to division or subtraction.
When adding or multiplying algebraic expressions, you may regroup the terms. This is called the associative property:
x + (y + z) = (x + y) + z x(yz) = (xy)z
Notice in these formulas that the variables have not been moved, only the way they are grouped has changed: on the left side of the formulas the last two variables are grouped together, and on the right side of the formulas the first two variables are grouped together.
For example, (x -2x) + 5x = (x + [-2x]) + 5x = x + (-2x + 5x) = x + 3x = 4x and 24x = 2x(12x) = 2x(3x4x) = (2x3x)4x = 6x4x = 24x
Caution: the associative property doesn’t apply to division or subtraction.
Notice in the first example that we changed the subtraction into negative addition: (x – 2x) = (x + [- 2x]). This allowed us to apply the associative property over addition.
Order of Operations: (PEMDAS)
When simplifying algebraic expressions, perform operations within parentheses first and then exponents and then multiplication and then division and then addition and then subtraction. This can be remembered by the mnemonic:
PEMDAS Please Excuse My Dear Aunt Sally
GRAPHS
Questions involving graphs rarely involve any significant calculating. Usually, the solution is merely a matter of interpreting the graph.

1. During which year was the company’s earnings 10 percent of its sales?
(A) 85
(B) 86
(C) 87
(D) 88
(E) 90
Reading from the graph, we see that in 1985 the company’s earnings were $8 million and its sales were $80 million. This gives 8/80 = 1/10 = 10/100 = 10%. The answer is (A).
2. During what two-year period did the company’s earnings increase the greatest?
(A) 85-87
(B) 86-87
(C) 86-88
(D) 87-89
(E) 88-90
Reading from the graph, we see that the company’s earnings increased from $5 million in 1986 to $10 million in 1987, and then to $12 million in 1988. The two-year increase from ’86 to ’88 was $7 million — clearly the largest on the graph. The answer is (C).
3. During the years 1986 through 1988, what were the average earnings per year?
(A) 6 million
(B) 7.5 million
(C) 9 million
(D) 10 million
(E) 27 million
The graph yields the following information:
| Year | Earnings |
| 1986 | $5 million |
| 1987 | $10 million |
| 1988 | $12 million |
Forming the average yields
(5 + 10 + 12)/3 = 27/3 = 9
The answer is (C).
4. If Consolidated Conglomerate’s earnings are less than or equal to 10 percent of sales during a year, then the stockholders must take a dividend cut at the end of the year. In how many years did the stockholders of Consolidated Conglomerate suffer a dividend cut?
(A) None
(B) One
(C) Two
(D) Three
(E) Four
Calculating 10 percent of the sales for each year yields
| Year | 10% of Sales (millions) | Earnings (millions) |
| 85 | .10 x 80 = 8 | 8 |
| 86 | .10 x 70 = 7 | 5 |
| 87 | .10 x 50 = 5 | 10 |
| 88 | 10 x 80 = 8 | 12 |
| 89 | .10 x 90 = 9 | 11 |
| 90 | .10 x 100 = 10 | 8 |
Comparing the right columns shows that earnings were 10 percent or less of sales in 1985, 1986, and 1990. The answer is (D).
WORD PROBLEMS
Although exact steps for solving word problems cannot be given, the following guidelines will help:
1) First, choose a variable to stand for the least unknown quantity, and then try to write the other unknown quantities in terms of that variable.
For example, suppose we are given that Sue’s age is 5 years less than twice Jane’s and the sum of their ages is 16. Then Jane’s age would be the least unknown, and we let x = Jane’s age. Expressing Sue’s age in terms of x gives Sue’s age = 2x – 5.
2) Second, write an equation that involves the expressions in Step 1. Most (though not all) word problems pivot on the fact that two quantities in the problem are equal. Deciding which two quantities should be set equal is usually the hardest part in solving a word problem since it can require considerable ingenuity to discover which expressions are equal.
For the example above, we would get (2x – 5) + x = 16.
3) Third, solve the equation in Step 2 and interpret the result.
For the example above, we would get by adding the x’s: 3x – 5 = 16. Then adding 5 to both sides gives 3x = 21. Finally, dividing by 3 gives x = 7. Hence, Jane is 7 years old and Sue is 2x – 5 = 2(7) – 5 = 9 years old.
Motion Problems
Virtually, all motion problems involve the formula Distance = Rate x Time, or
D = R x T
Example : Scott starts jogging from point X to point Y. A half-hour later his friend Garrett who jogs 1 mile per hour slower than twice Scott’s rate starts from the same point and follows the same path. If Garrett overtakes Scott in 2 hours, how many miles will Garrett have covered?
(A) 2 1/5
(B) 3 1/3
(C) 4
(D) 6
(E) 6 2/3
Following Guideline 1, we let r = Scott’s rate. Then 2r – 1 = Garrett’s rate. Turning to Guideline 2, we look for two quantities that are equal to each other. When Garrett overtakes Scott, they will have traveled the same distance. Now, from the formula D = R x T, Scott’s distance is D = r x 2 1/2 and Garrett’s distance is D = (2r – 1)2 = 4r – 2. Setting these expressions equal to each other gives 4r – 2 = r x 2 1/2. Solving this equation for r gives r = 4/3. Hence, Garrett will have traveled D = 4r – 2 = 4(4/3) – 2 = 3 1/3 miles. The answer is (B).
Work Problems
The formula for work problems is Work = Rate x Time, or W = R x T. The amount of work done is usually 1 unit. Hence, the formula becomes 1 = R x T. Solving this for R gives R = 1/T.
Example : If Johnny can mow the lawn in 30 minutes and with the help of his brother, Bobby, they can mow the lawn 20 minutes, how long would take Bobby working alone to mow the lawn?
(A) 1/2 hour
(B) 3/4 hour
(C) 1 hour
(D) 3/2 hours
(E) 2 hours
Let r = 1/t be Bobby’s rate. Now, the rate at which they work together is merely the sum of their rates:
Total Rate = Johnny’s Rate + Bobby’s Rate
1/20 = 1/30 + 1/t
1/20 – 1/30 = 1/t
(30 – 20)/(30)(20) = 1/t
1/60 = 1/t
t = 60
Hence, working alone, Bobby can do the job in 1 hour. The answer is (C).


